Algebraic fields

Overview and definition

Division ring

A ring with unity $R$, where every element has a multiplicative inverse, is a division ring or skew field if:

• $R\ne \{0\}$ as a group under addition is nontrivial: Equivalently, the additive and multiplicative identities $1\ne 0$ are not equal;
• Every nonzero element of $R$ has a multiplicative inverse: Equivalently, the group of units is $R^{\ast }=R\backslash \{0\}$.

A field is a commutative division ring.

In general, to show that a set $R$ is a field, we show that $(R,+)$ is an abelian group and that multiplication is associative, commutative, and distributes over addition.

Relevant theorems:

• (Theorem) Existence of a primitive root
• (Theorem) Classification of finite fields

Related notes:

• Finite extension fields

---

Properties

Fields and homomorphisms

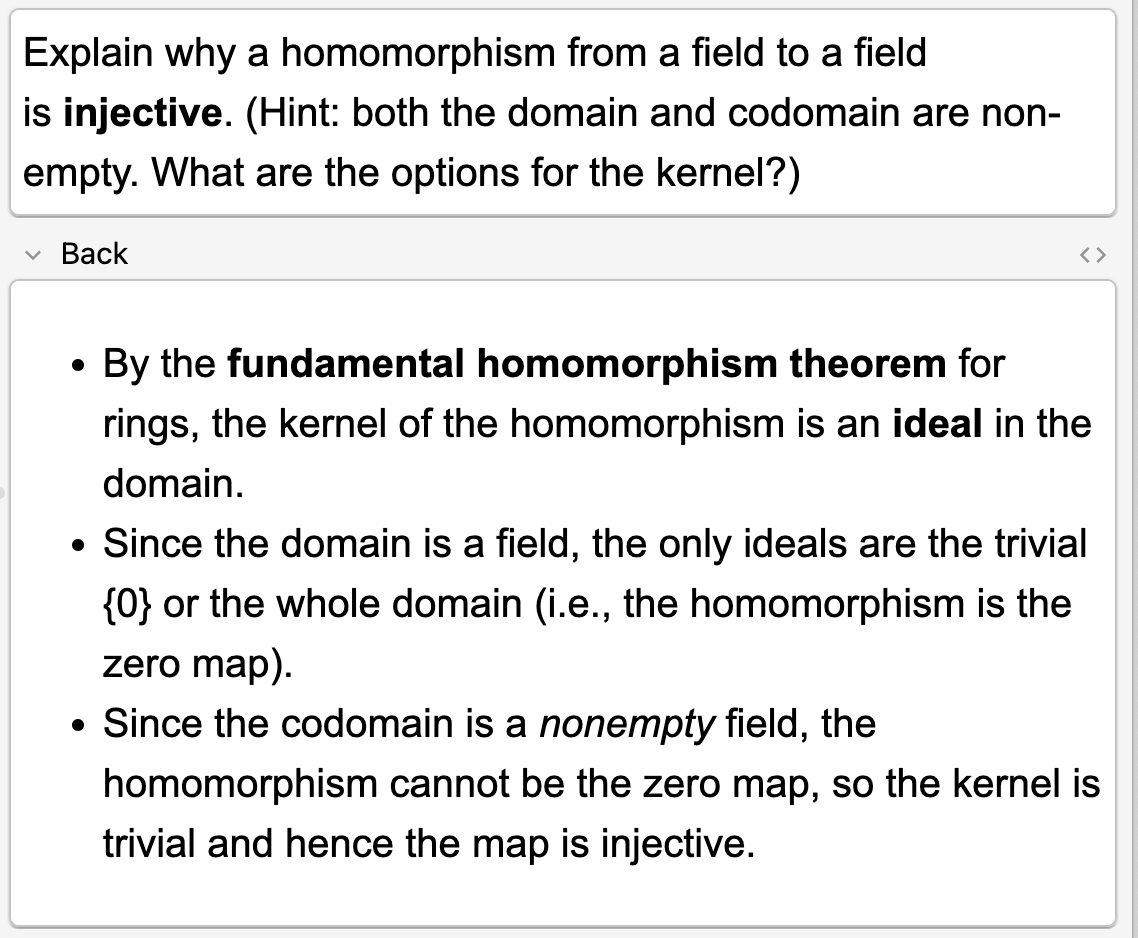
• Modern Algebra II, HW 3.1: If $\phi :F\to R$ is a homomorphism from a field $F$ to any ring $R$, then either $\phi$ is injective or $R=0$. In particular, if $R$ is also a field, then $\phi$ is injective.

• Ideals and quotient rings: Fields have no proper nonzero ideals, meaning the only ideals of a field $F$ are $\{0\}$ and the entire field $F$.

Finite fields

• Polynomial roots: F finite means eval hom is never injective, but it is always surjective

• (Theorem) Existence of a primitive root: If $F$ is a finite field, then the multiplicative group of units $(F^{\ast },\cdot )$ is cyclic.

• (Theorem) Classification of finite fields:

• Perfect fields: Every finite field is perfect, since the Frobenius homomorphism $\alpha \mapsto {\alpha }^p$ will be surjective on the finite set and hence an isomorphism; this makes every element into a $p$th power.

• Splitting fields: If ${\mathbb{F}}_p$ for prime $p$ and $q=p^n$ for some $n\in \mathbb{Z}$, then the splitting field of the polynomial $x^q-x$ over ${\mathbb{F}}_p$ is ${\mathbb{F}}_q$.

• Separable, normal, and Galois extensions of fields: If $p$ is prime and $q=p^n$, then ${\mathbb{F}}_q$ is a separable

• wip

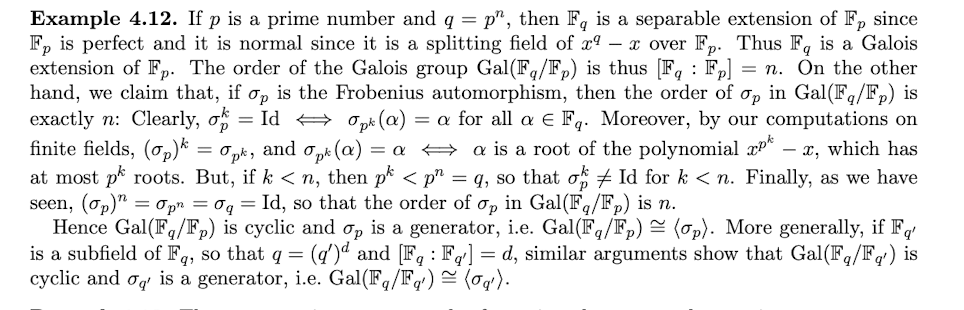
Polynomials with coefficients in $F$

• Ideals and quotients in polynomial rings: Every ideal in $F[x]$ is a principal ideal.